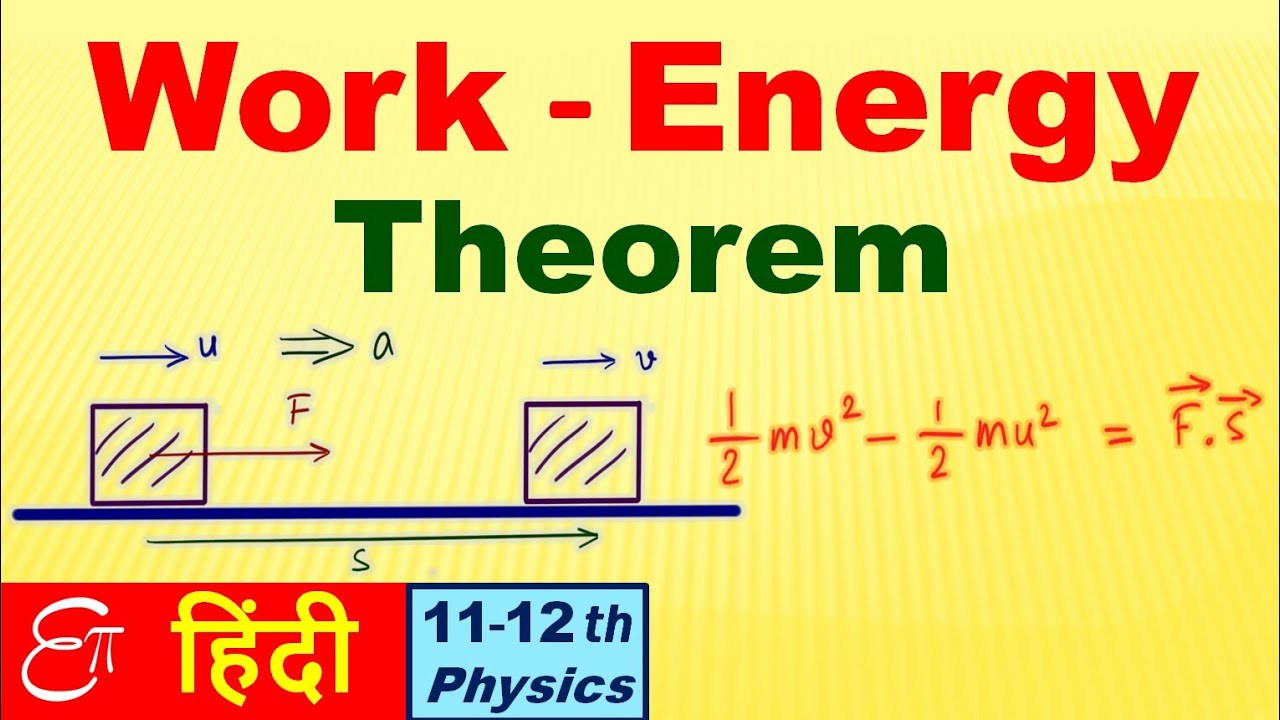
The work energy theorem also known as the principle of work and kinetic energy states that the total work done by the sum of all the forces acting on a particle is equal to the change in the kinetic energy of that particle.
Work energy theorem formula derivation.
To develop a mathematical expression that relates work to the kinetic energy we ll assume that all of the work done on a system gives the system kinetic energy only.
This is the derivation of work energy theorem.
For the sake of simplicity we will consider the.
The kinetic energy derivation using only algebra is one of the best ways to understand the formula in depth.
We will start with the definition of work and then apply newton s second law.
The work done on an object is equal to the change in its kinetic energy.
A variable force is what we encounter in our daily life.
The work energy theorem is useful however for solving problems in which the net work is done on a particle by external forces is easily computed and in which we are interested in finding the particles speed at certain positions of even more significance is the work energy theorem as a starting point for a broad generalization of the concept.
The net work w done on a particle during a given time interval by the net force acting on the particle is equal to the change in the particle s kinetic energy during.
Derivation of the work kinetic energy theorem for a single particle.
Workdone under a variable force.
The work w done by the net force on a particle equals the change in the particle s kinetic energy ke.
Derivation of the mathematical expression for work kinetic energy theorem.
Thus we can say that the work done on an object is equal to the change in the kinetic energy of the object.
Work energy theorem for variable force.
A constant force is rare in the everyday world.
Starting with the work energy theorem and then adding newton s second law of motion we can say that.
Latex text w delta text ke frac 1 2 text mv text f 2 frac 1 2 text mv text i 2 latex where v i and v f are the speeds of the particle before and after the application of force and m is the particle s mass.
General derivation of the work energy theorem for a particle.
The force that we come across everyday is usually variable forces.
Deriving the work energy formula for variable force is a bit hectic.
Therefore we have proved the work energy theorem.
For any net force acting on a particle moving along any curvilinear path it can be demonstrated that its work equals the change in the kinetic energy of the particle by a simple derivation analogous to the equation above.
The work kinetic energy theorem for a single particle.
Work kinetic energy theorem for a system of particles.